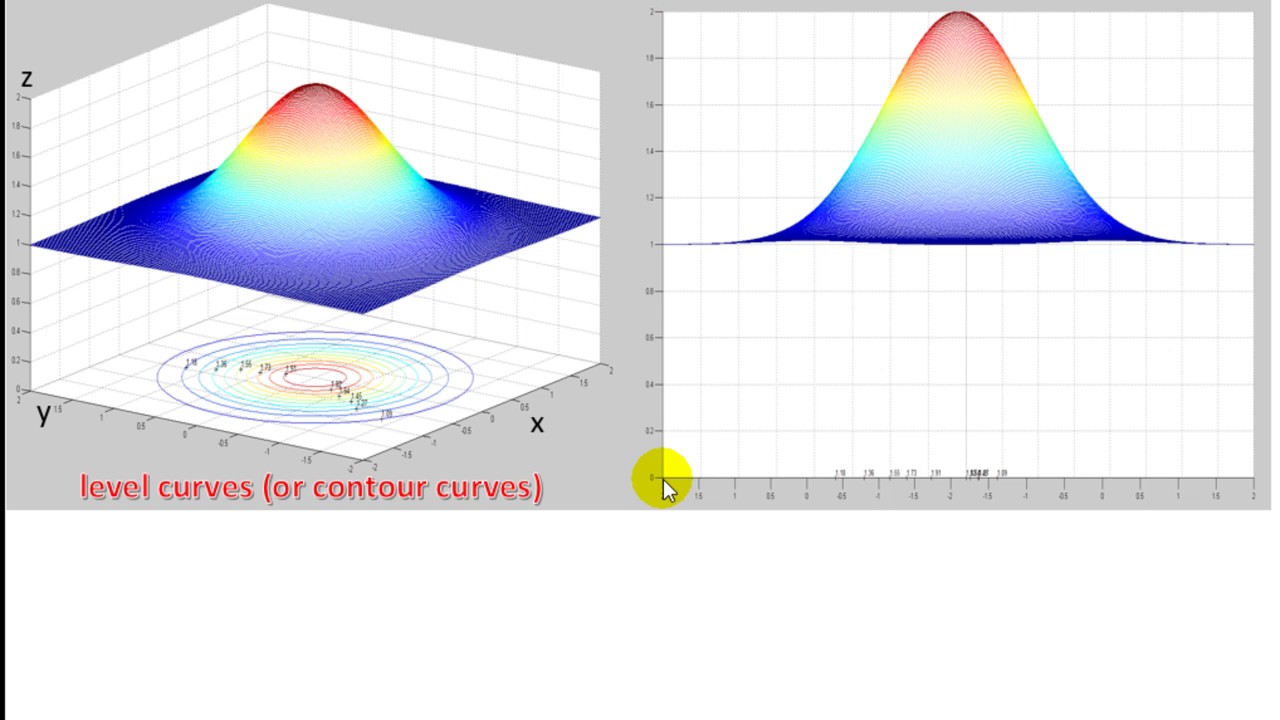
A graph of (some of) the level curves of a function is called a contour plot (it looks like a topographical map) Example Let's plot of the surface and several horizontal planes The curves of intersection are level curves (or contours) at levels and 1An Aframe level is a simple tool that allows us to easily find contours on the land, enabling us to build garden beds that harvest rainwater and flow with the landscape Building an Aframe level is easy, and can be done with scrap materials Get two long pieces of wood approximately the same length;Ie the level curves of a function are simply the traces of that function in various planes z = a, projected onto the xy plane The example shown below is the surface Examine the level curves of the function

Mitres 18 001 Guide13 Matematica
Level curves function contour lines
Level curves function contour lines-A Contour line may be defined as an imaginary line passing through the points of equal devotion A contour line may also be defined as the intersection of a level surface with the surface of the earth When the contours are drawn underwater, they are termed as Submarine Contours, Fathoms or Bathymetric CurvesKeep in mind at any given point (x, y), there are infinite directions we could move Each affects f (x, y) differently Just two directions don't affect it at all those are the contour line directions When we move perpendicularly to the contour line, that direction changes f (x, y)



14 Partial Derivatives Ppt Download
The projection of the contour line at height c into the xyplane is called the level curve at level c Thus a level curve is a plane curve Since the level curves of a function are implicitly defined plane curves, they can be drawn with the use of implicitplot But aWith the default settings Exclusions>Automatic and ExclusionsStyle>None, ContourPlot breaks continuity in its sampling at any discontinuity curve it detects The discontinuity is immediately visible only if it jumps out of a particular contour level Possible settings for0 Level curves allow to visualize functions of two variables f(x,y) Example For f(x,y) = x2 − y2 the set x2 − y2 = 0 is the union of the lines x = y and x = −y
Highlight Specific Contour Levels This example shows how to highlight contours at particular levels Define Z as the matrix returned from the peaks function Round the minimum and maximum data values in Z and store these values in zmin and zmax, respectively Define zlevs as 40 values between zmin and zmax Plot the contour linesA level set is a generalization of a contour line for functions of any number of variables Contour lines are curved, straight or a mixture of both lines on a map describing the intersection of a real or hypothetical surface with one or more horizontal planesCreate contour lines For this, we select CivilCAD>
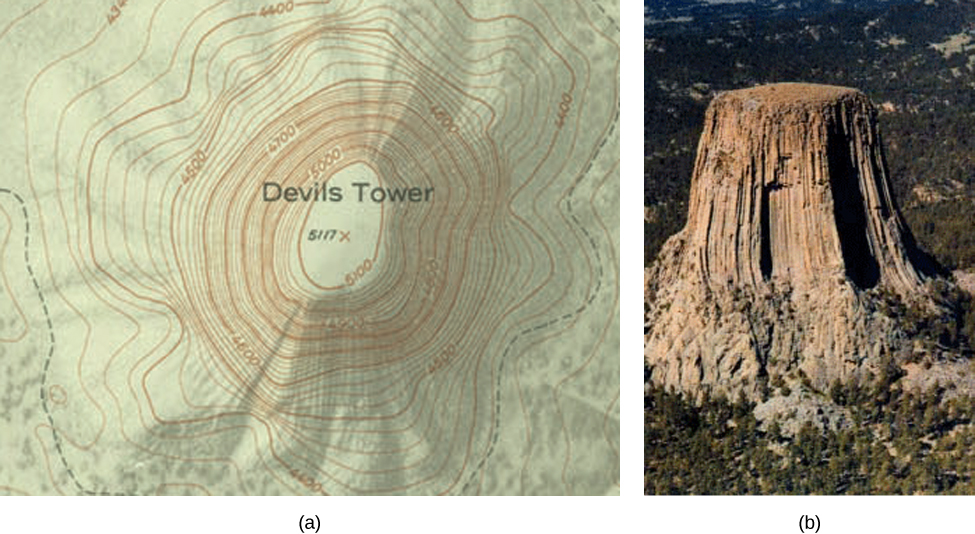
How to recreate contour lines from an existing contours?A contour is a line drawn on a map that joins points of equal height above sea level For 125 000 scale maps the interval between contours is usually 5 metres, although in mountainous regions it may be 10 metres How contour lines show a pair of small hillsThese maps are marked with contour lines or curves connecting Topographic (also called contour) maps are an effective way to show the elevation in 2D maps These maps are marked with contour lines or curves connecting The level curves are parallel lines of the form \2xy1=c\ The graph and some level curves are drawn in Figure 5



2



Contour Lines Or Level Curves
Level Curves In this activity we will introduce Matplotlib's contour command, which is used to plot the level curves of a multivariable function Let's begin with a short discussion of the level curve concept Hikers and backpackers are likely to take along a copy of a topographical map when verturing into the wilderness (see Figure 1)Contour Lines are rows of trees planted level along a hill's contour They intersect rainwater runoff, trap eroding soil, and build terraces of fertility over time <See What are Contour Lines?>A contour line (also known as a level curve) for a given surface is the curve of intersection of the surface with a horizontal plane, z = c A representative collection of contour lines, projected onto the xyplane, is a contour



Level Curves Or Contour Curves Youtube




Session 25 Level Curves And Contour Plots Part A Functions Of Two Variables Tangent Approximation And Optimization 2 Partial Derivatives Multivariable Calculus Mathematics Mit Opencourseware
33 Representation with contour lines an algorithm problem Although determining the color of a pixel by interpolation is easy, constructing the contour lines sensu stricto is more difficult Indeed, a contour line is an equation curve f(x, y) = C k, with C k being the kth level1 Consider the function f ( z) = z 2 Prove that level curves of R e ( f ( z)) and I m ( f ( z)) at z = 1 2 i are orthogonal to each other I am not sure how to apply level curves or contour lines for complex variables As far as real variables go, I am aware that for a function like f ( x, y) = x 2 y 2, the level curves are the circlesVisit wwweconomicswithfranciscocom (English) wwweconomiaycienciacom (Spanish)Level Curves or Contour Curves that will help to unders



14 Partial Derivatives Ppt Download



Level Curves Functions Of Several Variables By Openstax Page 3 12 Jobilize
Plot contour lines Call signature contour( X, Y, Z, levels, **kwargs) contour and contourf draw contour lines and filled contours, respectively Except as noted, function signatures and return values are the same for both versions Parameters X, Yarraylike, optional The coordinates of the values in ZContour lines are used eg in geography and meteorology In cartography, a contour line joins points of equal elevation (height) above a given level, such as mean sea level We can also say in a more general way that a contour line of a function with two variables is a curve which connects points with the same valuesSketch the level curves (contour lines) of the function?




Session 25 Level Curves And Contour Plots Part A Functions Of Two Variables Tangent Approximation And Optimization 2 Partial Derivatives Multivariable Calculus Mathematics Mit Opencourseware




Function Reference Contour
Ie the level curves of a function are simply the traces of that function in various planes z = a, projected onto the xy planeWhat is Contour line in Surveying ?Any size will do, but 1×2″ seem to be the most ergonomic



Level Curves And Contour Plots Mathonline



1


